< List of probability distributions > Bingham distribution
The Bingham distribution can be used to model the distribution of data points on a sphere. It is especially useful for modeling the local behavior of any smooth distribution around a stationary point and is frequently used in directional statistics. Its applications include computer vision [1], differential privacy [2] and paleomagnetic data analysis [3].
Bingham distribution properties
The Bingham distribution probability density function (PDF), with respect to the uniform (surface) measure is defined by a matrix A ∈ ℝd x d and given as [4]
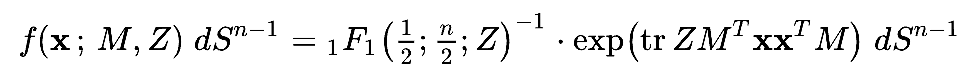
The matrix A defines the direction of the distribution, and the uniform (surface) measure — which assigns equal weight to every point on the surface of a d-dimensional sphere — ensures that all directions are equally likely. This means that the probability of a point being chosen from the surface of the sphere is the same for all points on the surface.
The more general Fisher-Bingham distribution includes a linear term, which means it can locally model any smooth probability distribution. Any smooth probability distribution resembles a Bingham distribution in a sphere of small radius around a stationary point. Thus, the Bingham can be thought of as a “model” non-log-concave distribution [5]. Non-log-concave distributions such as the Cauchy distribution and Pareto distribution can’t be represented as the product of a concave function and a non-negative function. They tend to be more challenging to work with than log-concave distributions, but they can model a wider range of phenomena such as multimodal distributions, including those that are also skewed.
Bingham Distribution extensions
Various extensions of the Bingham have been formulated including the general eight-parameter Fisher-Bingham distribution family on the sphere, first proposed by Kent [6] .
The general form of the Fisher-Bingham (FB) distribution is given by the partial differential equation
C exp [κ0(x′ξ0) + κ1(x′ξ1)2 – κ2(x′ξ2)2] dS
Where ξ is a unit vector (ξ1, ξ2 are at right angles and involve five parameters) and κ is a shape parameter. The distribution can be unimodal or bimodal depending on the values of the shape parameter; it can also be concentrated around a closed curve.
- The FB4 is called the Bingham-Mardia distribution after Christopher Bingham and K.V. Mardia [7].
- The FB5 is usually called the Kent distribution, based on Kent [6].
- The FB6 distribution was first introduced in Rivest [8].
References
[1] M Antone and Seth Teller. Automatic recovery of camera positions in urban scenes. Technical
Report, MIT LCS TR-814, 2000.
[2] Kamalika Chaudhuri, Anand D Sarwate, and Kaushik Sinha. A near-optimal algorithm for differentially-private principal components. The Journal of Machine Learning Research, 14(1): 2905–2943, 2013.
[3] Tullis C Onstott. Application of the Bingham distribution function in paleomagnetic studies. Journal of Geophysical Research: Solid Earth, 85(B3):1500–1510, 1980.
[4] Christopher Bingham. An antipodally symmetric distribution on the sphere. The Annals of Statistics,
pages 1201–1225, 1974.
[5] Ge, R. et al. Efficient sampling from the Bingham distribution. Proceedings of Machine Learning Research vol 132:1–13, 2021 32nd International Conference on Algorithmic Learning Theory
[6] Kent, J. (1982). The Fisher-Bingham Distribution on the Sphere. Journal of the Royal Statistical Society. Series B (Methodological). Vol 44, No.1, pp.71-80. Wiley.
[7] Bingham & Mardia. A small circle distribution on the sphere. Biometrika, Volume 65, Issue 2, August 1978, Pages 379–389
[8] Rivest, L. On the Information Matrix for Symmetric Distributions on the Hypersphere.